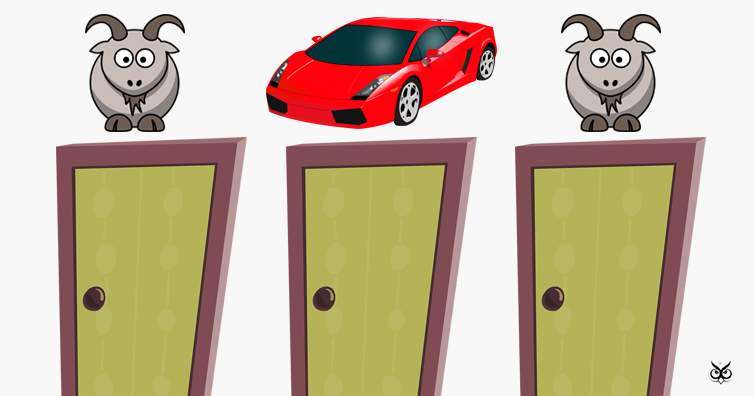
Imagine you are on a game show. The game show host shows you three doors. Behind one of the doors, is the star prize; a brand new car. Behind the other two doors are booby prizes; two goats. Whichever door you choose you’ll receive the price behind it.
You are asked to pick a door. but before it is opened, the game show hosts opens one of the other doors. Now, the host knows where the car is and he always opens a door to reveal a goat. You are then asked whether you’d like to swap your chosen door for the one remaining closed door. The question is: should you swap, should you stick with your original choice or does it make no difference at all what you do? Which will give you the greatest chance of winning the car?
Most people will say that it makes no difference whether you swap or not. Behind one closed door is a goat and behind the other closed door is the car, therefore the chances of choosing the car are 50/50. This sounds perfectly sensible, however it’s not correct. The Monty Hall problem is a puzzle about probability and even though is simple to understand, the answer is counterintuitive. So what should you do? (the article continues after the ad)
The answer is you should always swap as this gives twice the chance of winning the car. Why? Well, even though there are many ways to explain why, perhaps the easiest is to examine what your chances of winning the car are for the two strategies: swapping and not swapping. Let’s see what happens what when you choose not to swap
NOT SWAPPING
At the start of the game you are asked to pick a door. Since there are 3 doors and only 1 hides the car, the probability of you picking the car is 1/3 or about 33%. Since there are 2 goats, the probability of you picking a goat is 2/3 or about 66%. If you don’t swap your door it doesn’t matter which other door the host opens because you are sticking with your first choice, hence the chance that you’ve already picked the car is 33% and a goat 66%. So, by not swapping you have:
- 33% chance of winning the car
- 66% chance of winning a goat
SWAPPING
Now let’s look at the consequences of swapping. Let’s consider that by luck, you pick the car first time – a 33% percent chance. It’s obvious that if you picked the car on your first go and then swap you are going to end up with a goat. So if you swap, you are going to end up with a goat 33% of the time.
But what about if you picked a goat first time? Well, here is the crux of the problem. This time, there is only one goat the host can reveal. The host opens the only other goat door and then you swap to the remaining closed door – the car. In fact, every time you pick a goat door first time and then swap, you will win the car. And the chances of you picking a goat first time are 66%, so by swapping you have:
- 66% chance of winning the car
- 33% chance of winning a goat
So, you should always swap to the remaining door because if you do, you’ll double your chances of winning the car and half the chance if you don’t.
Does this makes sense to you? Do you see the logic behind the Monty Hall problem?
If you like what you read, then you will definitely love this one: Is Zero An Even, An Odd Number Or Neither?
Photo: OpenClipart-Vectors / Pixabay, Open Clip Art Library, Wikimedia
Photoshop: I’m A Useless Info Junkie
Sources: The Monty Hall Problem